Research Topics
Nachfolgend finden Sie 3 ausgewählte Forschungsthemen.
Directed-Loop Quantum Monte Carlo Method for Retarded Interactions
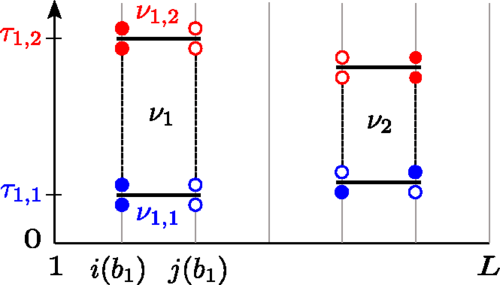
The directed-loop quantum Monte Carlo method is generalized to the case of retarded interactions. Using the path integral, fermion-boson or spin-boson models are mapped to actions with retarded interactions by analytically integrating out the bosons.
This yields an exact algorithm that combines the highly efficient loop updates available in the stochastic series expansion representation with the advantages of avoiding a direct sampling of the bosons.
The application to electron-phonon models reveals that the method overcomes the previously detrimental issues of long autocorrelation times and exponentially decreasing acceptance rates. For example, the resulting dramatic speedup allows us to investigate the Peierls quantum phase transition on chains of up to 1282 sites.
Zero Modes and Global Antiferromagnetism in Strained Graphene
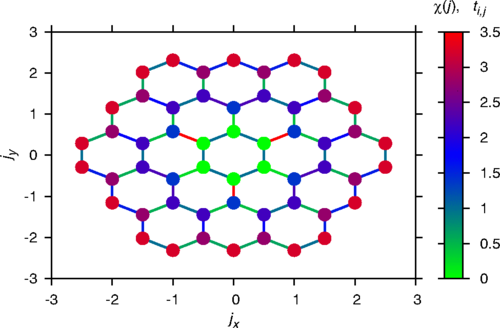
A novel magnetic ground state is reported for the Hubbard Hamiltonian in strained graphene. When the chemical potential lies close to the Dirac point, the ground state exhibits locally both the Néel and ferromagnetic orders, even for weak Hubbard interaction.
Whereas the Néel order parameter remains of the same sign in the entire system, the magnetization at the boundary takes the opposite sign from the bulk. The total magnetization vanishes this way, and the magnetic ground state is globally only an antiferromagnet. This peculiar ordering stems from the nature of the strain-induced single-particle zero-energy states, which have support on one sublattice of the honeycomb lattice in the bulk, and on the other sublattice near the boundary of a finite system.
We support our claim with the self-consistent numerical calculation of the order parameters, as well as by the Monte Carlo simulations of the Hubbard model in both uniformly and nonuniformly strained honeycomb lattice. The present result is contrasted with the magnetic ground state of the same Hubbard model in the presence of a true magnetic field (and for vanishing Zeeman coupling), which is exclusively Néel ordered, with zero local magnetization everywhere in the system.
Dimerized Solids and Resonating Plaquette Order in SU(N)-Dirac Fermions
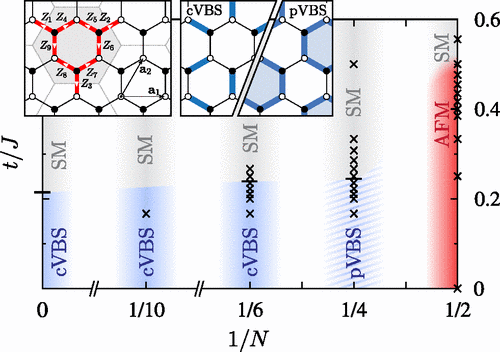
We study the quantum phases of fermions with an explicit SU(N)-symmetric, Heisenberg-like nearest-neighbor flavor exchange interaction on the honeycomb lattice at half filling. Employing projective (zero temperature) quantum Monte Carlo simulations for even values of N, we explore the evolution from a weak-coupling semimetal into the strong-coupling, insulating regime.
Furthermore, we compare our numerical results to a saddle-point approximation in the large-N limit. From the large-N regime down to the SU(6) case, the insulating state is found to be a columnar valence bond crystal, with a direct transition to the semimetal at weak, finite coupling, in agreement with the mean-field result in the large-N limit.
At SU(4) however, the insulator exhibits a subtly different valence bond crystal structure, stabilized by resonating valence bond plaquettes. In the SU(2) limit, our results support a direct transition between the semimetal and an antiferromagnetic insulator.