Recent Research Topics
Nachfolgend finden Sie 3 ausgewählte aktuelle Forschungsthemen.
Quantum Spin Hall Effect (in Science, mit F. Reis, G. Li et. al., June 29 (2017))
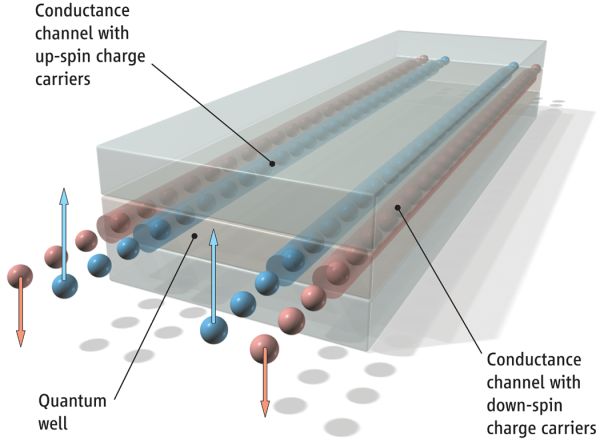
Quantum-Spin-Hall (QSH) materials are two-dimensional representatives of the family of topological insulators. They promise revolutionary device applications ("spintronics") based on dissipationless propagations of spin currents at their edges (see Fig. above).
After the detection of the celebrated Quantum-Hall state by K. von Klitzing in Würzburg 1980, for which he was rewarded the Nobel Prize in 1985, the QSH effect was again first realized in Würzburg by L. Molenkamp´s group 2007.
However, in all QSH systems realized so far, the small bulk energy gap (~30meV) prevents applications at room temperature. In our very recent Science letter, we present combined theoretical and experimental work on Bismuthene on a SiC Substrate, establishing this composite system as a prime candidate for a new room-temperature QSH material.
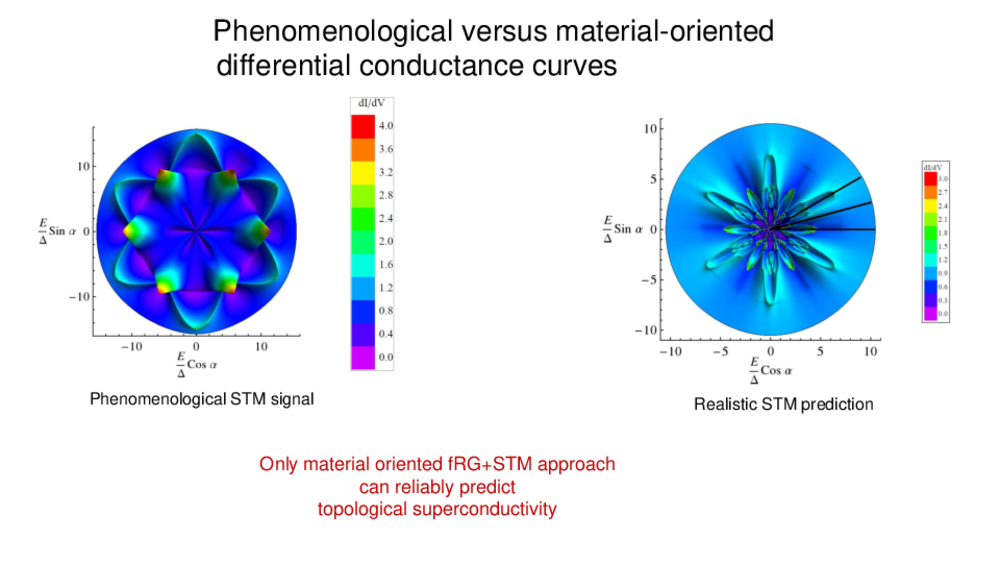
Accessing topological superconductivity via a combined STM (scanning-tunneling-microscopy) and renormalization-group analysis (Nature Comm. 6, 8283 (2015))
The search for topological superconductors (TSC) has recently become a key issue in condensed matter physics, because of their possible relevance to provide a platform for Majorana bound states, non-Abelian statistics and quantum computing.
With L. Elster et. al, Nature Comm. 6, 8232 (2015), we propose a new scheme which links as directly as possible the experimental search (i.e. the powerful scanning tunnelling microscopy (STM)) to a material-based microscopic theory for topological superconductivity (multi-orbital functional renormalization group analysis).
For paradigmatic systems such as sodium cobaltates, we demonstrate that our microscopie material-oriented procedure is necessary to uniquely resolve a topological "d+id", see Fig. above, superconducting state.
Exploring the World of Interacting Fermions with the Functional Renormalization Group (Review in Adv. in Physics, Vol 62, 453 (2013))
When one wants to resolve the competing phases (such as the anti-ferromagnetic (AF) and superconducting (SC) phases in e.g. the High-Temperature Superconductors) one has to bridge in a controlled manner an enormous difference in energy scales, or in temperatures:
The constituent microscopic particles are at "high energy" i.e. the interactions of the electrons with themselves and with the ions are typically of the order of eV´s. However, the crucial competing phases appear at several orders of magnitude smaller energy scales, i.e. meV´s, or, at low temperatures. The idea of the functional RG method is precisely to bridge these scales via systematically "integrating out" the high-energy degrees of freedom.
In our Review (Adv. in Phys. 67, 453 (2013)) on the functional RG, we present both methodology progress (multi-orbital systems) and applications to key issues, such as new (topological) quantum states of matter, in particular predictions of topological SC phases.